Marvelous Math Transcript

Welcome

This is Susan C. Anthony speaking.  Welcome to my Marvelous Math workshop for teachers and homeschoolers.  This is one of my favorite workshops because most adults learn something new themselves, in addition to learning new ways to teach and review basic calculation.

About Me

I’ll tell you a little about myself so you’ll know who’s talking.  This photo is of me in a boat at our homestead in Interior Alaska.  My husband homesteaded in 1964 and it’s our little piece of heaven on earth.  I’ve always loved wilderness and adventure.  I grew up in the mountains of Colorado, where our parents let explore without supervision for hours, as long as we were home for dinner.  It’s probably more dangerous there now than then.  There are cougars and bears now, as well as a lot more people.

I was an overachiever in school.  I got 100% on every single Algebra I paper and test, then got a D in Algebra II.  My first-year Algebra II teacher had never taught before and the texts were horrible.  They were confusing and had lots of misprints.

I like math because I like clarity.  Answers are black or white, right or wrong.  Whether you get a wrong answer because you don’t know the concept or you made a careless error, it’s wrong, period.  Today I’ll show you ways to improve kids’ accuracy and diligence in basic calculation, which builds their confidence.

Objectives

My objectives today are to:

• Build a good foundation for math instruction.
• Share the best math tricks I know.  Hopefully, teach you something in math that you don't already know.
• Boost your confidence in the area of math.
• Equip you to get kids enthused about math.

One thing that alarmed me as a 6th grade teacher was that kids came to me seeming to know or remember very little from their previous years of math instruction.  I always had to review for a couple of months before we could move on.  One year all the 6th grade teachers in our school met with junior high teachers to learn what we could do better to prepare kids for Junior.  They said PLEASE teach kids to write both first and last names on their papers, and in math, if we could just make sure all the kids knew how to add, subtract, multiply and divide, they could teach everything else.  Even in Junior high, teachers had to spend an inordinate time reviewing.

I’ll show you some fun ways to review basic math today.  You may find yourself in a situation where you have no alternative, so might as well make it fun.

Handouts

A handout is available to download from my web site, SusanCAnthony.com. If you’re listening to this presentation online, you should print out at least the first page of the handout because I’ll ask you to do the practice problems on the bottom as we go along.

The second page of the handout gives details about the Gelosia method of multiplication, which is one thing I’ll show you.

The third and fourth pages explain casting nines, which is the best method I ever found for raising the accuracy of student computation.  Kids enjoy it, so checking their work becomes less of a drag and more of a game.

The Problems

• Kids "know" the math, but make careless mistakes.  In math, wrong is wrong, period.  Careless mistakes were the bane of my existence as a math teacher.
• Too much math is taught too soon without regard for mastery.
• Student resistance.  Kids had "had" it. 
• It's difficult to erect a structure on a bad foundation.

When I moved from teaching 6th grade to teaching 4th grade, I was shocked to see that the math texts were almost identical.  Same march of chapters, with a couple extra at the end of the 6th grade texts.  Maybe that was part of the problem.   Kid had “had” a concept even although they didn’t know it.  They were resistant.  That’s how immunization works.  People are injected with a little bit of virus so their body can build resistance.  When kids are resistant, teaching can be a challenge.

My Mom sent me an article from the Denver Post while I was preparing this workshop.  According to it, in order for kids to be ready for algebra in high school, they need to master these things in the elementary grades.

• 1st Grade:  Addition and subtraction through 20.
• 2nd Grade:  Addition and subtraction of 3-digit numbers; counting by 2s and 3s.

First and second grade requirements seem reasonable, but look at third grade!

• 3rd Grade:  Multiplication of 3 and 4-digit numbers, short division, beginning long division.
• 4th Grade:  Long division, beginning word problems, fractions, decimals and percentages.
• 5th Grade:  Conversion of percentages to fractions, beginning geometry.
• 6th Grade:  Word problems, decimals, percentages, positive and negative numbers, algebraic expressions.

Remember back to what those junior high school teachers said.  They wanted kids who could add, subtract, multiply and divide.  Anything else is bonus!  Their problem was they had to spent months reteaching basic math before they could move on!

Original Krypto

Most of what I did well in teaching math I learned from my husband.  His students loved this game, Krypto.  He wrote a Krypto problem on the board every morning before kids came in for them to work on while they waited for class to begin.  Whoever got a a correct answer won a round of applause.

Just an aside here, I taught kids in my class to applaud each other by clapping their hands 7 times fast and then stopping.  I don’t want desultory applause and I don’t want any time to be wasted.

In Krypto, you first shuffle the cards, then lay out five cards in a row with one below.  The challenge is to combine the five numbers on the top using addition, subtraction, multiplication and division in order to get the number on the bottom.

Here’s an example.  The challenge is to combine 6, 1, 20, 7 and 8 in some way to get five.  Give it a try without looking at the answer.

Here is one possible answer.

1. Subtract one from six to get five.
2. Divide the 20 by that five (from #1) to get 4.
3. Add the 4 (from #2) to the 8 to get 12.
4. Subtract the 7 from the 12 (from #3) to get 5.

Here is another possible answer, combining the five cards in a different way to get 5.

1. Subtract the 7 from the 8 to get 1.
2. Subtract the 1 from the 6 to get 5.
3. Divide 20 by 5 (from #2) to get 4.
4. Add 4 (from #3) to the 1 to get 5.

There can be several ways of combining the numbers to get the target number, which is what makes it fun and challenging.  It helps kids learn to use mental flexibility in math.

Here’s a Krypto problem for you to try.  Combine the five numbers on the top to equal 10.

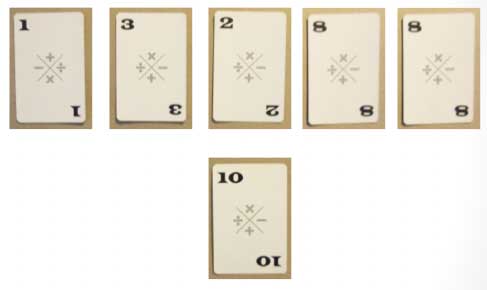
Here are a couple of ways that work.

1. Add 1 + 3 + 8 + 8 to get 20.
2. Divide 20 by the 2 to get 10.

And another way.

1. Add the 3 and the 2 to get 5.
2. Subtract the 8 from the 9 to get 1.
3. Add that 1 (from #2) to the first card’s 1 to get 2.
4. Multiply the 5 (from #1) and the 2 (from #3) to get 10.

Hopefully, you get the idea.

Place Value

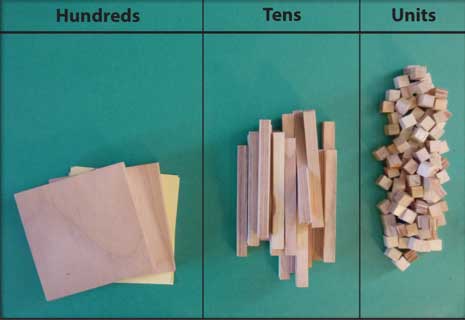
Manipulatives are popular for teaching math, but can be kind of costly.  I found that I only needed two class sets of manipulatives to teach just about anything I needed to teach.  One was Base 10 blocks.  I highly recommend you get a set of base 10 blocks for each child you teach.

These are lined up according to place value, with the unit (a small cube) on the far right, a rod (representing 10) to its left, a flat representing 100 and a large cube representing 1000.

To introduce these,

1. Hold up a unit cube and define it as a unit, or "one."
2. Put ten cubes side by side and place a ten rod next to them.  Notice they're the same length.  Define the rod as a "ten."
3. Put ten rods side by side and place a flat on top of it.  Notice that they're the same quantity.  Define the flat as 100.
4. Stack 10 flats on top of one another and show that the 1000 cube is the same size.  Notice also that it's the same shape as the unit cube, but a lot larger.

I kept a Ziplock bag for each child in a box in the classroom.  Each bag contained 100 small unit cubes, 21 ten rods, and 2 hundred flats, with pieces of paper the size of the hundreds to represent additional hundreds.  To the right is a thousand cube

After doing what I just described, I piled 10 of these on top of each other to show the value of 10,000.  Here’s my sleepy husband in his bare feet keeping my 10,000 from toppling over and breaking something.

Notice this is the same shape as a 10 rod, but a whole lot bigger.

I never had enough blocks to show 100,000 but I wanted to.  I photoshopped 10 of the 10,000 stacks together here.  Notice that it's the same shape as the hundred flat, only a whole lot bigger.  I thought it would be fun to get dowels or something and at least show the space a million cube would occupy, but I never got around to it. 

This exercise wowed the kids and provided a good foundation for teaching place value.

To teach place value, make a chart like this on a 12 x 18 piece of paper for each child.

Model how to assemble pieces to represent various numbers.  For example 246.  Place two flats in the 100’s column, four rods in the tens column, and six ones in the unit’s column.  Say or write other numbers.  Lay out pieces and have the kids write the number represented.

Work into zero as a place holder.  The number 204, for example, would have one flat in the hundreds column, three cubes in the units column, and NOTHING in the tens column.

Do this with a variety of numbers until the kids are very good at it.

Once kids understand place value, I give them a chart like this every day to fill out from right to left, like this.

Ones, tens, hundreds, one thousands, ten thousands, hundred thousands, one millions, ten millions, hundred millions, one billions.  Notice that each grouping consists of ones, tens and hundreds.  I call the groupings “families.”  The families are units, thousands, millions, billions, and so on.  My book Facts Plus lists families all the way to decillions. 

So the sequence for teaching place value, a concept foundational to everything in math is:

• Initially teach place value with Base 10 blocks.
• Students fill in one place value chart every day (ones, tens, hundreds, one thousands, ten thousands, hundred thousands, etc.
• Teacher dictates numbers.  Students write them in the grid and on the line to the right, with commas in proper places.
• Students practice reading large numbers (you can find large numbers in Facts Plus and other reference books.
• Dictate large numbers with zeros and have students write them.
• Have students round large numbers they've written.

Later on, you can use the same blocks to teach decimals.  Show kids how the little cube and the thousand cube are the same shape.  To get a tenth of the small unit cube, you’d have to saw it into ten equal pieces.  To get 100th, you’d have to saw each of those into 10 equal pieces, and 1000th would be teeny tiny.  (I always thought it would be fun to have examples to show and pick up with tweezers.)

Obviously, pieces like that would be too small to work with, so we’re going to redefine the larger cube as a unit instead of 1000.  1/10th of the large cube is a flat.  1/100th of the large cube is a rod, and 1/1000th is a small cube.

Make a chart on 12 x 18 paper, similar to before.

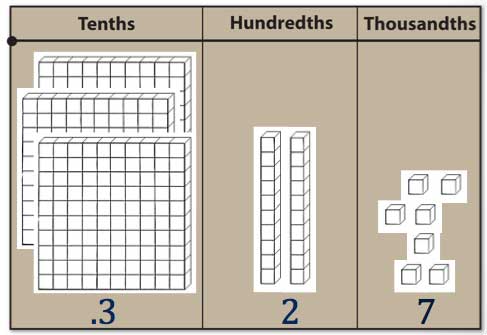
Here’s the decimal place value chart they can fill in day after day until they have it memorized, as with the place value chart I showed earlier.  Use this the same way.  Once kids can fill it in correctly every time, say a number and have them write it.  Work into numbers with zeros as place holders. Expand little by little until kids can write any number you say and round it.  Note that in this illustration the names of the places are filled in. Give kids blank charts so they fill them in.

Learning Math Facts

My math-facts-in-five-minutes-a-day system is to give all kids an opportunity to memorize all math facts to MASTERY at their own rate.  Some kids zoom through this and then work to better their times.  Others take all year.  I’ve had kids come back in the day after school ended when I was cleaning up the classroom to take one last test, pass it, and earn a Susan B. Anthony dollar, real money, as a reward.  It was well worth it to give each child a dollar of my own money for memorizing their facts to mastery.

Each child gets a folder with a sticker sheet taped in on the left and a Record of Timed Tests taped in on the right.  I usually fill in all the dates school will be in session before reproducing the Record of Timed Tests.  We do this activity just about every day all year.  It takes only a few minutes a day.

I also include colored paper with answer keys for all the tests.

I also put a chart on the wall showing all the kids’ names and all the tests.  When a child passes a level, I let him or her put a star on that chart.  When kids’ parents came to visit, they’d often drag them right over to the chart.

The first day everybody does a worksheet with just zeros and ones. Believe it or not, this covers 21 of the 66 basic facts.  A lot of kids pass this the first day or two and move on to twos.  From there, they start to diverge according to ability.  Some kids move on to threes before others do.  Everybody practices in the same few minutes of time, but everybody is working at their own level.  After memorizing ones, twos, and threes, they get a worksheet that mixes 0s to 3s, then 4s, 0s to 4s, all the way to tens.

There are five different tests that mix all of the facts, so they rotate through them until they can get all 100 in three minutes or less.  There are lots of variations you can do, but that’s the basic idea.

Some people say kids shouldn’t have to master their facts because we have calculators, but who pulls out a calculator to multiply 5 x 4?  By mastering their facts, they’ll gain confidence as well as be better prepared to learn more advanced math.  The facts should become background information, so well known that if I shook a child half awake at 3:00 a.m. and asked “what’s 8x7, he’d answer correctly and fall right back to sleep.”  I want them to know these facts as well as they know their own names.

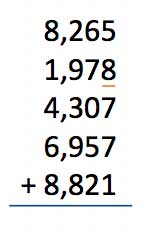Adding by Endings

Now I’ll show you something that a Mensa member friend of mine told me was the most useful thing he learned in all of elementary school, adding by endings.

To add by endings, start at the top of the units column.  I insist kids touch the numbers with their pencils and say them aloud.  Say “5 + 8 is 13.”  What is the ending of 13?  3.  Right.  Now keep the 3 in your head and put a little line underneath the 8 to represent the 10 from 13.  Notice the little red line.  It represents the 10 in 13. The 3 is still in your head.

Now move on to add the 3 you kept in your head to the next number in the column. Say 3 + 7 = 10. What is the ending on ten (0).  So this time I keep a zero in my head.  What do I do with the 10?  (Draw a line, right.)

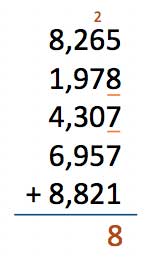Move on by adding the zero in your head to the next number in the column, which is a 7.  That’s just one digit so keep it in your you your head and go on.  Don’t draw a line unless you have two digits. 

Add the 7 in your head to the last number in the unit’s column which is one, 7 + 1 = 8, then write an 8 under the units column, like is shown to the right:

Now count the lines you made in that column and carry that number.  You have a line under eight and a line under seven, so carry 2, like this.

Now start from the top to add the tens column.  The 2 that you carried plus 6 = 8, 8 + 7 = 15. 

What do I do?  Keep the 5 in my head and put a line under the 7.

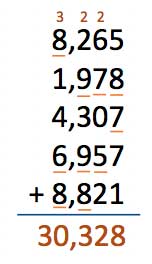Continue with  the five in your head 5 + 0 = 5,

5 + 5 = 10, keep the 0 in your head and put a line under the 5. 0 + 2 = 2 and 2 is what you write at the bottom.

How do you know how many to carry?  Count the lines in the column.  There are two, one under 7 and one under 5.  So carry 2. 2 + 2 = 4, 4 + 9 = 13 so keep the 3 in my head and draw a line under the 9.  3 + 3 = 6, 6 + 9 = 15 (draw a line).  5 + 8 = 13 (DRAW A LINE).  Be very careful at the bottom of a column!  Don’t be tempted to say 13, write the 3 and carry 1.  You have to count all the lines in the column and carry them.  In this case, there are three lines so carry 3.  Continue.  The whole problem is done correctly to the left.

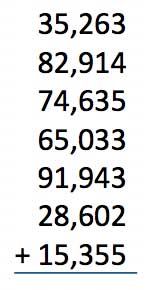
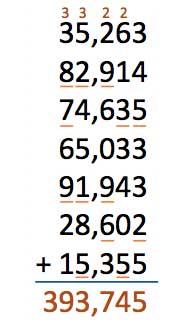Now you try it.  This long problem is on the handout.  I know you can get the answer your normal way, but just for fun, try the adding by endings way.

I recommend that you teach kids this method of column addition from the beginning.  It’s just as easy to do a problem like the one here using adding by endings as it is to do a much smaller problem.  Once kids complete a really big addition problemcorrectly, they gain confidence.  They know that they know that they know how to add.  You probably noticed that addition facts are all they need to know to be succeed, as long as they’re careful and methodical.

If you have older kids, like I did, that need to review and master addition, this is a way to make review more interesting.  I gave kids a worksheet with addition problems of all sizes—long, skinny ones, short squat ones, and a huge one like this.  Their assignment was to chose from the problems and do what they could in the time allowed. Some kids preferred to do several smaller problems, others went straight for the gold.  When anyone got a huge problem like this correct, I made a big deal out of it.  I called in the principal.  She projected the problem during an assembly and had the ones who had succeeded stand up for a round of applause. 

If kids lose their place on a problem like this, they should go back to the top of the column they didn’t finish and start there.  They should touch each numeral with their pencil as they move down the column.

I once had a second grader in a homeschool group who really didn’t like math.  I asked if I could show him how to do this.  He very reluctantly agreed.  He learned quickly and I gave him a huge problem like this to take home.  The next week he was back with the problem finished, asking if I had another one, please.

Gelosia Multiplication

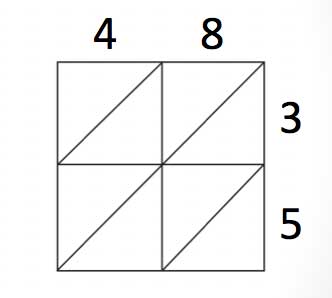The next trick I’ll show you is Gelosia multiplication, also known as lattice multiplication.  Note that I normally wouldn’t teach kids two or three things in a single day like this.  If your head is spinning with adding by endings, stop here and come back to learn this next thing some time later.  We're going to multiply 48 x 35.  The answer is 1,680.  This is a simple problem to do the regular way, but I want to use it to show that you get the same result using Gelosia multiplication.

Start with half-inch graph paper.  Draw a square or rectangle on the graph paper to fit your problem.  In this case you will multiply a 2-digit number by another 2-digit number, so you'll marke out a 2 x 2 square.

Congratulate yourself!  You're 1/3 done with the problem.

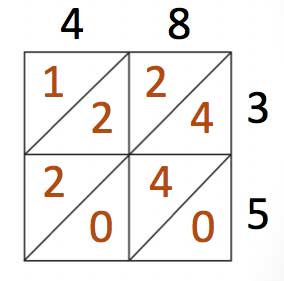
Next, fill in the lattice, as shown to the left.  4 x 3 = 12, so write that answer in as shown. 8 x 3 is 24, etc.

More congratulations are due.  You’re now 2/3 of the way to the answer!

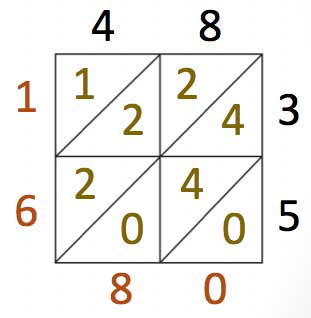
The final step is to add the columns diagonally as shown to the right.  Copy the numbers in a line and you get 1680. Add the comma and it's 1,680, the same answer you got doing the problem the regular way.

You wouldn't normally use Gelosia multiplication for such a small problem.  The beauty of it is you can do HUGE problems that would be very difficult the normal way.  Here's a bigger problem, 6,292 x 435.

The answer is to the right.

Copy the numbers: 2737020

Add the commas: 2,737,020.  That's the answer.

For large problems, it’s helpful to use adding by endings at least for the long middle columns so you don’t get confused. 

Like adding by endings, I used this to teach kids how to correctly do HUGE multiplication problems, problems that are as big as they want them to be.  I’ve had kids make up their own problems with multiplicand to the decillions along the top of a full sheet of half-inch graph paper and multiplier all the way down the side.  Whether or not they complete the whole huge problem is of less concern to me than the fact that they’re excited about multiplication and gaining confidence. 

The largest problem I generally ask them to try is 5 digits x 5 digits.  For example:  48,367 x 29,512.  But some kids love this and want to make up and complete the biggest problem of anyone in the class.

You probably noticed that they’re practicing multiplication facts again and again just by filling in the lattice. 

Casting Nines

The last thing I want to show you is casting nines.  This is confusingly similar to adding by endings, so as I said before, if you’ve learned enough for one day, stop now and come back later to learn this.  This workshop was created to be presented in a one-hour chunk of time, so that was a restraining factor.  Normally, especially in math, you want to proceed one small thing at a time and give kids LOTS of practice before introducing something new.

Casting nines is a way to check accuracy of computation.  It's "fun" and kids actually use it.  It's especially useful with very large numbers.  It's confusingly similar to adding by endings.  Teach this at a widely different time.  I found the best place to introduce this was after teaching fourth graders 2-digit x 2-digit multiplication, such as 48 x 35. 

First I'll show you how to cast nines, then I'll tell you why.  Please be patient.  Everybody always has the Why? question foremost in their minds as I show them how, so try to ignore that question for a minute.

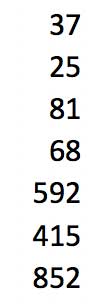Here is HOW you cast nines from a number.  Add the digits of the number.  For example, in 37, add the 3 + 7 to get10.  Then throw away any nines, 10 - 9 = 1.  The result of casting nines from 37 is 1.

Think about it.  If you divide 37 by 9 you get 4 with a remainder of 1.  All numbers are multiples of 9 with no remainder or with a remainder of 1,2,3,4,5,6,7,or 8.  You’re finding that residual, the remainder, what’s left over when all the nines are gone.

Look at the next one, 25.  Add the digits, 2 + 5.  The answer is 7.  There are no nines to throw away so when you cast nines from 25, you get 7.

Look at the next one, 81.  8 + 1 is 9, throw the nine away and you get 0.

I know your minds are screaming WHY do this?  Try to put that question aside.  I promise you’ll see why soon.

OK, 68.  6 + 8 = 14.  To cast nines, you can subtract 9 from 14 to get 5, OR, there’s an easier way.  If you end up with a 2-digit number like 14, just continue adding the digits of the number until you have only one left.  So instead of subtracting 9 from 14, add the 1 + 4 in 14 to get 5.  You get the same answer with an easier process. 

How about 592.  Well, you know you’re not going to need that 9, so throw it away to start with.  Add 5 + 2 and you get 7.

How about 415?  4 + 5 makes nine, so just throw those two digits away.  All that’s left is 1.

And the last one, 852.  To keep it easy, I’d add 8 + 2 which equals 10, get rid of the 9 leaving one, then add 1 + 5 to get 6.

The power of casting nines reveals itself when you have BIG numbers!  Remember, I’ll show you why in a minute. 

Look at the first one.  See any nines?  6 + 2 + 1 = 9, so when you cast nines you get 4.  I have kids put a little colored dot below digits they throw away so they don’t get confused and add them in somewhere.

Is there anything you can throw away in the next big number?  First throw away the nine.  Throw away 7 + 2, which equals nine. Throw away 8 and 1 which equals nine. Then add the 6 + 4 you have left, throw away the nine.  The result is 1.

Now it's your turn to try.  Cast nines from the numbers to the right.

The correct answers, in order, are 2, 3, 0, 8, 4, 0 and 8.  How did you do?

Checking Addition

You have been patient.  Now let’s answer the WHY question!

The purpose for casting nines is to check computation for accuracy.  I don’t know about you but the bane of my existence as a math teacher was careless errors.  A child could do a problem perfectly in front of me, yet on his own miss five of ten problems just like it.  Most kids aren’t naturally careful.  All my exhortations to pay attention and be careful fell on deaf ears.

It got to the point where I made my students do every math problem twice before moving to the next problem!  I definitely didn’t want kids practicing errors!  Practice doesn’t make perfect.  Perfect practice makes perfect.

So I’ll show you how casting nines works to check addition, then multiplication, and finally subtraction and division.  It is worth taking the time to teach casting nines because it has broad applicability and virtually eliminates the headache of careless errors.

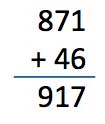We'll start with a simple problem, 871 + 46.  The first thing you do is cast nines from your addends.  Cast nines from 871 and you get 7.  Cast nines from 46 and you get 1.

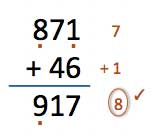Because this is an addition problem, you add those two digits, 7 and 1, to get 8.  Circle the 8.  It is your "check number." 

Now cast nines from your sum.  If the result is the same, 8 in this case, your answer is correct.  Good for you!  Put a check next to the check number to show it matched and move to the next problem. (Note that on the video I go through a couple of additional problems before students try one.

Now it’s your turn.  This problem is on the handout.  If you cast nines from 468, you get 0.  If you cast nines from 327, you get 3.  Add 0 + 3 and the check number is 3.  If you cast nines from 795, you get 3.  They match.  YEAH!  The sum is correct.

Checking Multiplication

Now let’s look at checking multiplication.  I tried introducing my 4th graders to casting nines early in the year, but most of them just got confused.  I backed off, happy enough that they understood the idea of adding by endings.

In January, when we started multiplying two digit by two digit numbers, careless errors skyrocketed.  I introduced casting nines again.  This time the kids got it, and they blazed on through multiplication without anymore big issues.

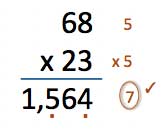It’s done the same as you did addition.  Cast nines from the multiplcand and multiplier, casting nines as you go, to get a one-digit check number.  Since it’s a multiplication problem, you multiply.  5 x 5 = 25.  Keep casting nines.  2 + 5 = 7.  So 7 is your check number.

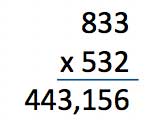Now cast nines from your product.  Notice that I tossed the 5 and the 4 right away.  You get 7 so the answer is RIGHT.  YEAH!

It's your turn.  Do the problem on the right.

When you cast nines from 833, you get 5.  When you cast nines from 532, you get 1.  Since this is a multiplication problem, multiply 5 x 1.  That equals 5, which is your check number. Cast nines from the product, 443,156 and you get 5. The answer is correct and you know it!

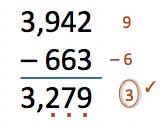Checking Subtraction

Cast nines from the minuend and the subtrahend to get the check number.  Since this is a subtraction problem, you subtract!  The problem is, sometimes you can't subtract.  In that case, add a nine to the top number so you can subtract.  See the example.

Now you try.  Do the problems below.  Remember to add a nine to the top number if needed so you can subtract.

When you cast nines from 692, you get 8.  When you cast nines from 325 you get 1.  8 - 1 = 7, the check number.  Now cast nines from the difference 367, to get 7.  It matches.  The answer is correct.

Now for the second problem, when you cast nines from 1,243, you get 1.  When you cast nines from 746, you get 8.  It is impossible to subtract 8 from 1, so add a 9 to the 1 to get 10, then subtract 8 to get 2, the check number.  Cast nines from 497 to get 2.  It matches.  The answer is correct.

Checking Division

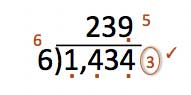To check division, you normally multiply the quotient (the answer) by the divisor (the number in front, to the left of the bracket).  The answer should equal the dividend (the number inside the bracket).

You do the same thing to check division using casting nines, but first you throw out all the nines.  In the example, nines have been cast from 239 to get 5, from 6 to get 6, and from 1,434 to get 3 (the check number).  The long way of checking would be to multiply 239 x 6, which should equal 1,434. With casting nines, do the same thing but with smaller numbers.  5 (from the 239) x 6 = 30.  Cast nines from the 30 and get 3.  That matches the check number.  The answer is correct!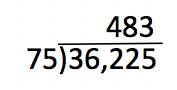

Now you try checking the division problem on the left. 

The check number is 0 and the answer is correct.

Putting It All Together

Once kids mastered these three tricks, adding by endings, gelosia multiplication, and casting nines, my 4th graders issued a challenge to a 6th grade class, with the winner to be the class with the highest average score on 10 basic problems.  Nothing special, just addition, subtraction, multiplication, and division.  The older kids said sure, why not, taking for granted they’d win.  The younger kids practiced and practiced on BIG problems.  The best 4th grade students helped kids who found this harder because every single correct answer contributed to the class average and every single wrong answer knocked it down.

The 4th graders won.  My husband’s brother had his 4th graders challenge 8th graders and they won, not because they were better mathematicians, but because with big problems, there are so many opportunities to make careless errors.  The key is to be careful and check your work.

Kids who’ve successfully solved BIG problems thereafter think the “regular” problems in their textbooks are simple.  When they’re members of a class that bests an older class on a class average, even if their individual paper was one of the poorest in the group, they gain confidence.  They like math better.  They start wanting to learn.  Your job gets easier.

For me, teaching the marvelous math tricks I shared with you today made a huge difference for lots of kids in their achievement and in their attitude toward math.  Confidence is a wonderful thing.

I’d love to hear any math success stories you have from using these ideas. e-mail me (Susan@SusanCAnthony.com).  And be sure to check my web site for more free math ideas in the Resources section, including a free booklet you can use to introduce fractions.

Source:  www.SusanCAnthony.com, ©Susan C. Anthony